Wastewater Treatment Plant Design...

Chapter 03 - Sedimentation...

3.1. Introduction...

There is usually a large fraction of organic material suspended in domestic wastewaters which does not fully obey simple relationships given in the previous chapter since it tends to coalesce or flocculate. This material is often removed in settlement tanks prior to secondary treatment. In addition, material of 70 - 80 % organic content is produced by the secondary processes and usually must be removed prior to effluent discharge.

3.2. Overflow Rates...

Consider a discrete particle which reaches a terminal velocity V O when settling in an ideal horizontal tank such as in figure given below line "a". Scouring is assumed not to occur, and particles which settle such that they just reach the invert prior to the end of the tank will be removed.



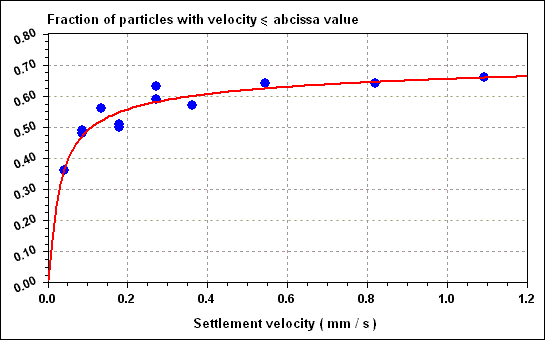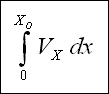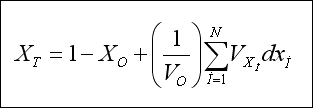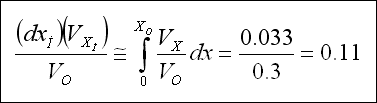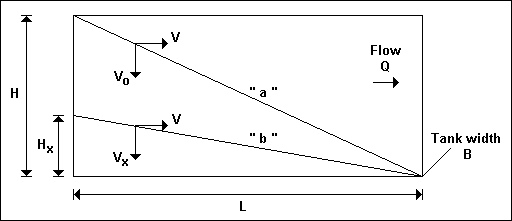
Q = ( V ) ( B ) ( H )

where ; Q : forward flow through the tank, H : depth, B : width and V : mean forward velocity assumed to be constant at all points in the the tank. By geometry ;

( V / V O ) = ( L / H )

V O = ( Q ) / [ ( L ) ( B ) ]

This settlement velocity is referred to as the design settlement velocity of the tank and can be used to predict settlement efficiencies within the tank. This velocity is also known as the surface loading rate or overflow rate of the tank.

Example 3-1 :

A flow of 20 L / s of a mixed suspension of discrete particles of relative density ( specific gravity ) of 2.60 is to be settled in an ideal settlement tank having a surface area of 5.00 m 2 . Assuming NU = 1.00 x 10 - 6 m 2 / s, calculate the diameter of particles, 100 % of which will be settled. Also, determine the effect of halving the depth.

Calculation :

V O = ( Q ) / [ ( L ) ( B ) ] = ( 20 x 10 - 3 ) / ( 5.00 ) = 4.00 x 10 - 3 m / s

d = { [ ( V O ) ( 18 NU ) ] / [ ( g ) ( R D - 1 ) ] } 1 / 2

d = { [ ( 4.00 x 10 - 3 ) ( 18 x 1.00 x 10 - 6 ) ] / [ ( 9.81 ) ( 2.60 - 1 ) ] } 1 / 2 = 6.8 x 10 - 5 m = 0.068 mm

Particles with relative densities of 2.60 with diameters than 0.068 mm will settle completely, some smaller particles will pass through the tank. If the depth is halved, the forward velocity V is doubled. However the particles have only half the original vertical distance to fall. Thus ;

( 2 V / V O ) = [ ( L ) / ( H / 2 ) ]

( V / V O ) = ( L / H )

Therefore, changing the depth has no effect unless extreme conditions of shear are produced, i.e. the tank ceases to be an ideal tank. Line " b " represents the path taken by a particle with settlement velocity V X which enters the tank at height H X in a homogeneous column of suspension of depth H. If it settles out in a tank of design settlement velocity V O , then by geometry ;

( H X / L ) = ( V X / V )

Substituting for V = ( L / H ) ( V O )

( H X / H ) = ( V X / V O )

Example 3-2 :

For the conditions given in Example 3-1, determine the propotion of particles with R D = 2.60 and diameter 0.04 mm which settle in the basin.

Calculation :

V X = [ ( g ) ( d 2 ) ( R D - 1 ) ] / ( 18 NU )

V X = [ ( 9.81 ) ( 4.00 x 10 - 5 ) 2 ) ( 2.60 - 1 ) ] / ( 18 x 1.00 x 10 - 6 ) = 0.0014 m / s

Therefore the proportion settling is ;

( V X / V O ) = ( 1.40 x 10 - 3 / 4.00 x 10 - 3 ) = 0.35 = 35 %

3.3. Batch Settlement Analyses...

A column of the type shown figure given below is filled with the heterogenous suspension, no particle of which has a velocity great enough to settle a distance > z 1 prior to the initial sampling. Small samples are taken at each outlet at set times and the suspended solids concentration in the samples is determined. If the original concentration in the column is C O at time t O = 0 and some time later is C I at time t = t I in a sample taken from depth z I , then C O - C I of the original suspension has settlement velocities >= ( z I ) / ( t I - t O ). When repeated for a range of z I and t I a series of values for C I is obtained and a cumulative curve of proportion of particles with velocity <= the abcissa value can be plotted.



Example 3-3 :

The results of a batch settlement analysis carried out on a mixed suspension are given in table shown below. Plot the cumulative velocity profile of the suspended solids.

Number Sample depth ( m ) Sample time ( h ) SS ( mg / L )
1, 2, 3 1, 2, 3 0 222 ( averaged )
4 1 1 140
5 1 3 108
6 1 6 80
7 2 1 142
8 2 3 110
9 2 6 106
10 3 1 142
11 3 3 130
12 3 6 124
13 4 1 147
14 4 3 126
15 4 6 114

Calculation :

Consider depth 2 m at time 3 h. The velocities of particles found in the sample are <= ( 2,000 ) / ( 3 x 3,600 ) mm / s, i.e. 0.19 m / s. Therefore ( 110 / 222 ) ( 100 % ) of particles have a settlement velocity < 0.19 mm / s. By a similar process we can construct table given below.

Number Velocity ( mm / s ) SS remaining ( fraction )
4 0.278 0.63
5 0.093 0.49
6 0.046 0.36
7 0.556 0.64
8 0.185 0.50
9 0.093 0.48
10 0.833 0.64
11 0.278 0.59
12 0.139 0.56
13 1.110 0.66
14 0.370 0.57
15 0.185 0.51

Since the fraction of particles remaining in a sample is the same as the fraction of particles which velocity less than or equal to the value of the velocity pertaining to that sample, the values in table given above may be used to construct the cumulative settlement velocity curve shown in figure given below.



The next step is to determine two fractions of particles ; ( a ) those whose velocity is greater than a chosen design settlement velocity V O and consequently will settle out, and ( b ) the fraction of those particles, which, although possessing velocities less than a chosen design settlement velocity V O will nonetheless contribute to the settled solids in the ratio V X / V O . For the weight fraction X O ( i.e. whose settlement velocities V X are <= V O ) the proportion which will settle X S is given by ;



The value ;



is the area bounded by the ordinate ( vertical axis ) and the curve between 0 and X O . For a chosen value of V O the total settleable solids X T in a tank with design settlement velocity V O is ;





where ; N : total number of segments.

Example 3-4 :

For design settlement velocities ( V O ) in the range 0.2 to 0.8 mm / s find the predicted percentage settlement of particles for the previous batch settlement test.

Calculation :

Inserting a value of V O = 0.3 mm / s on figure given above shows that a fraction X O = 0.58 of particles have a velocity <= V O . Thus 1 - X O in the third equation is 1 - 0.58 = 0.42. Other calculations were given table shown below.

dx I region dx I value Vx I ( dx I ) ( Vx I )
0.58 - 0.50 0.08 0.22 0.0176
0.50 - 0.40 0.10 0.10 0.010
0.40 - 0.30 0.10 0.04 0.004
0.30 - 0.20 0.10 0.015 0.0015
0.20 - 0.10 0.10 < 0.005 Neglected
0.10 - 0.00 0.10 < 0.005 Neglected
Total ( dx I ) ( Vx I ) = 0.0331

Thus ;



The total predicted settlement is given by ;



Similarly, by choosing other values of V O , figure given below may be constructed. In practice, the overflow rates at maximum flow are often 0.34 to 0.68 mm / s. For the sample described above, little theoretical removal efficiency is gained by using the lower value of 0.34 mm / s.


3.4. Upward Flow Tanks...

If a particle exists in an ideal upward flow tank with cross - sectional area A and overflow rate Q then the liquid upward flow velocity has a magnitude Q / A or V O ( the design settlement velocity ) as before. The resultant of V O acting vertically upwards and the settlement velocity of the particle V X will be upward if V X < V O ; zero if V X = V O and downwards if V X > V O . The total removal for a suspension would be ;

X T = 1 - X O

Example 3-5 :

For the suspension tested in the previous section, determine the percentage removal of particles expected in an upward flow tank with an overflow rate of 26 m 3 / m 2 . day.

Calculation :

Neglecting any liquid removed when desludging the design settlement velocity is ;

V O = ( 26 x 10 3 ) / [ ( 24 ) ( 3,600 ) ] = 0.3 mm / s

From the batch settlement analysis given in figure shown above, the value of X T = 1 - X O would be 1 - 0.58 as a fraction or 42 %. This compares with 53 % for a horizontal flow tank.

3.5. Flocculent Particles...

If a particle or mass of particles form transient ( weak ) or permanent ( strong ) bonds with each other they are said to flocculate. Where particles flocculate they increase their diameter with time of settlement. However in the case of flocculent removals it is more common to present the results in the form of a grid showing percentage removal of particles at particular retention times and depths similar to that given in figure shown below.



Example 3-6 :

Assuming the figures given below, calculate the theoretical percentage removal expected in a 2 m deep tank after 1 h retention and in a 2.5 m deep tank and 3.75 h retention.

Calculation :

From figure given above, the removal at 2 m depth and 1 h is seen by inspection to be 34 %. For 2.5 m and 3.75 h, arithmetic interpolation may be used, i.e.

% Removal = ( 1 / 2 ) [ ( 64 + 62 ) / ( 2 ) ] + [ ( 60 + 68 ) / ( 2 ) ] = 63.5 %

3.6. Choice of Tank Shape...

Typical operating criteria for settlement tanks in a temperate climate at maximum hourly flow rates, e.g. 3 DWF are given in table shown below.

Function Retention time
( h )		 Loading rate
( m 3 / m 2 . day )		 Weir overflow rate
( m 3 / m . day )		 Solids loading
( kg / m 2 . day )
Primary settlement
Large scale for activated sludge 1.0 - 1.5 30 - 45 < 220 -
Large scale for trickling filter 1.5 - 2.0 30 - 45 < 220 -
Small scale > 1.5 - - -
Humus tanks
Large scale 1.3 - 1.6 35 - 40 < 220 -
Small scale > 4.0 - - -
Activated sludge
Large scale - 45 - 60 < 250 < 180
Small scale - < 22 < 100 < 100

3.6.1. Radial Flow Tanks...

Figure given below shows a circular settlement tank with major dimensional relationships specified. These, together with the information from table given above can be used to determine tank dimensions.



Example 3-7 :

Calculate the approximate dimensions and number of tanks required to treat a maximum flow of raw sewage of 0.40 m 3 / s.

Calculation :

If we assume a peripheral ( single ) weir loaded at say 150 m 3 / m . day and the effective surface area of the tank ( tank area less inlet area ) to be loaded at 30 m 3 / m 2 . day, then the maximum individual tank diameter can be found by equating ( surface loading x area ) to ( weir loading x length ). An allowance is made in the diameter d to allow for the inlet area, i.e. ;

[ ( 30 ) ( PI ) ( d - 0.15 d ) 2 ] / ( 4 ) = ( 150 ) ( PI ) ( d )

d = ( 150 x 4 ) / ( 30 x 0.85 2 ) = 27.7 m

which is equivalent to a flow of ;

( 30 x PI x 27.7 2 x 0.85 2 ) / ( 4 x 8.64 x 10 4 ) = 0.15 m 3 / s

The number of tank required is therefore 3. The actual diameter of the 3 tanks is given by ;

( 3 x PI / 4 ) ( 0.85 2 ) ( d 1 2 ) ( 30 ) = ( 0.4 x 8.64 x 10 4 )

d 1 = 26.0 m

To obtain a retention time of say 2 h at peak flow, the average depth, D, would be ;

( 3 x PI / 4 ) ( 0.85 2 ) ( 26.0 2 ) ( D / 0.4 ) = ( 2 x 3,600 )

D = 2.5 m

This would be satisfactory for a wall depth. If the bottom slope was 10 O the actual retention time would be slightly greater than 2 h. The weir overflow rate ( WOR ) would be ;

WOR = ( 0.4 x 8.64 x 10 4 ) / ( 3 x PI x 26.0 ) = 140 m 3 / m . day

Assuming an inlet concentration of solids of, say, 200 mg / L ( 0.2 kg / m 3 ) and 60 % removal in the form of a sludge of 97 % moisture content ( 30 kg / m 3 ), the size of a sludge hopper sufficient to hold 12 h sludge production would be ;

V SLUDGE HOPPER = ( 0.4 x 8.64 x 10 4 x 0.20 x 0.6 ) / ( 3 x 2 x 30 ) = 23.0 m 3

The volume of the cone whose apex is 60 O is given by ;

V SLUDGE HOPPER = ( PI / 3 ) ( r B 2 ) ( h )

where ; r B : the hopper base ( tank floor ) radius and h : the hopper ( or cone ) depth. Since r B / h = tan 30 O , then ;

V SLUDGE HOPPER = 1.81 x r B 3

If the cone is truncated, with a bottom radius of 0.80 m then ;

1.81 x r B 3 - 1.81 x 0.80 3 = 23.0

r B = 2.4 m

It should be noted that radial flow tanks usually have wall depths of 2.5 - 3.5 m for primary settlement and 3.0 - 4.0 m for secondary settlement. Tank diameters of up to 60 m have been used for primary settlement ; however, they require an extra ( inboard ) weir and greater excavation. Bottom slopes between 5 O and 10 O are commonly used where scraper mechanisms are employed.

3.6.2. Rectangular Tanks...

Rectangular tanks are often used for primary settlement. They are not commonly chosen as activated sludge tanks since the efficient collection and return of such sludges is easier a radial flow continuously scraped tank. For rectangular tanks common ratios and values of length, depth and width are given in table shown below.

L : h = 12 : 1 ; L < 90 m
L : B = 4 : 1
h = 3.0 - 3.5 m ; maximum 5.0 m
t = 1.5 to 2.0 h at maximum flow
Minimum number of tanks = 2

Example 3-8 :

Calculate approximate sizes for primary settlement tanks to treat a maximum flow of 0.50 m 3 / s, using a design settlement velocity of 0.45 mm / s and a retention time ( at maximum flow ) 1.75 h.

Calculation :

Surface loading rate = ( 0.45 x 8.64 x 10 4 ) / ( 1,000 ) = 39 m 3 / m 2 . day

The total area required = ( 0.50 ) / ( 0.45 x 10 - 3 ) = 1,110 m 2

Tank volume = ( 0.50 x 3,600 x 1.75 ) = 3,150 m 3

Depth = ( 3,150 ) / ( 1,110 ) = 2.84 m ; say 3.00 m allowing for sludge storage

Length ( L ) : width ( B ) ratio = 4 ( assumed )

Area of 1 tank = ( 1,110 ) / ( n ) ( n is the tank number )

A 1 = ( 1,110 ) / ( n ) = ( B 1 ) ( L 1 )

A 1 = ( 1,110 ) / ( n ) = ( L 1 / 4 ) ( L 1 )

L 1 2 = ( 4 x 1,110 ) / ( n )

( Column - 2 ) L 1 = [ ( 4 x 1,110 ) / ( n ) ] 1 / 2
( Column - 3 ) B 1 = L 1 / 4
( Column - 4 ) Weir overflow rate ( WOR ) = ( Q ) / [ ( n ) ( B 1 ) ]
( Column - 5 ) Forward velocity ( FV ) = ( Q ) / [ ( n ) ( B 1 ) ( D 1 ) ]


n L 1
( m )		 B 1
( m )		 WOR 1
( m 3 / m . day )		 FV 1
( m / s )
2 47.1 11.8 1,830 7.1 x 10 - 3
3 38.5 9.6 1,500 5.8 x 10 - 3
4 33.3 8.3 1,301 5.0 x 10 - 3
5 29.8 7.5 1,152 4.4 x 10 - 3
6 27.2 6.8 1,058 4.1 x 10 - 3

The decision as to the number of tanks depends on the site confitions ; however, reducing the number of the tanks generally reduces the duplication of mechanical scraping equipment while increasing the weir overflow rate. If 4 tanks 33.3 m x 8.3 m were chosen the WOR would be 1,301 m 3 / m . day. Although some argument is common regarding the necessity of reducing this value to 200 m 3 / m . day, the former figure is clearly too high. Installing two double sided inboard weirs supported across the tank would, together with the end weir, reduce the WOR to 260 m 3 / m . day.

Example 3-9 :

Using the Clement's approach, design horizontal flow settling tanks for primary sewage ( scouring velocity 32 mm / s ). Maximum flow is 0.10 m 3 / s, V O is to be 0.60 mm / s and T R = 0.60.

Brief explanation of Clement's method :

- Mean forward velocity ( V ) = Scouring velocity / 8
- Time ratio factor ( T R ) = 0 ( for infinitely poor tank ) and 1 ( for ideal tank )
- ( L 2 ) / [ ( B ) ( D ) ] > 20

Calculation :

V = ( 32 ) / ( 8 ) = 4.00 mm /s

( V / V O ) = ( 4.00 / 0.60 )

( L ) / ( D ) = 6.7

A = ( Q MAX ) / [ ( T R ) ( V O ) ] = ( 0.10 ) / [ ( 0.60 ) ( 0.60 x 10 - 3 ) ] = 278 m 2

If B O = ( n ) ( B ), i.e. B O is the total width of n individual tanks of width B ( m ) ; then from constructional considerations ( L ) / ( B O ) should be range from 1 / 2 to 1. The surface area is ( L ) / ( B O ) ; thus L should be range from ( 278 / 2 ) 1 / 2 m to ( 278 )sup> 1 / 2 m, i.e. 11.8 m to 16.7 m. B O is therefore between 278 / 16.7 m and 278 / 11.8 m, or 16.6 m and 23.6 m. Since ;

( L 2 ) / [ ( B ) ( D ) ] > 20 then [ ( n ) ( L 2 ) ] / [ ( B O ) ( D ) ] > 20

and since L / D is set at 6.7 ;

[ ( n ) ( L ) ] / ( B O ) > 3.0

Values of tank dimensions for various values of L are given in the table shown below. If the values of n and B O corresponding to the particular choice of L are plotted as in figure shown below, 3 points are possible where the number of tanks is an integer value, i.e. ;

n = 3, B O = 16.7 m, L = 16.6 m, L / B = 3.0
n = 4, B O = 19.4 m, L = 14.3 m, L / B = 3.0
n = 5, B O = 21.6 m, L = 12.9 m, L / B = 3.0

L 12.0 13.0 14.0 15.0 16.0 17.0
B O 23.2 21.3 19.8 18.5 17.4 16.3
D 1.80 1.94 2.09 2.24 2.39 2.54
n 5.8 4.9 4.2 3.7 3.3 2.9
n ( rounded ) 6 5 5 4 4 3
B ( based on rounded n ) 3.9 4.3 4.0 4.6 4.4 5.4
L / B 3.1 3.0 3.5 3.3 3.6 3.1





The final choice could be made on site conditions, however, since increasing the tanks also increases the equipment cost, there is a tendency to choose 3 tanks, 16.6 m long and 5.5 m wide. The values of L / B have been included since values significantly less than 3.0 tend to reduce the values of T R and thus produce a penalty by increasing the value of ( L ) ( B O ).

3.7. Settlement Efficiency - Primary Tanks...

Tebbutt has proposed an equation to calculate the fractional removal efficiency E in a primary settlement tank with overflow rate Q / A and with influent suspended solids concentration ;

E = ( 0.955 ) exp - [ ( 265 / S I ) + ( 0.0021 ) ( Q / A ) ]

Example 3-10 :

Using equation given above determine the expected removal efficiency ( a ) for an overflow rate of 25 m 3 / m 2 . day and S I values of 300 and 600 mg / L, and ( b ) for an S I value of 400 mg / L, for overflow rates of 25 and 100 m 3 / m 2 . day.

Calculation :

( a ) For Q / A = 25 m 3 / m 2 . day and S I = 300 mg / L

E = ( 0.955 ) exp - [ ( 265 / 300 ) + ( 0.0021 ) ( 25 ) ] = 0.37

For Q / A = 25 m 3 / m 2 . day and S I = 600 mg / L

E = ( 0.955 ) exp - [ ( 265 / 600 ) + ( 0.0021 ) ( 25 ) ] = 0.58

( b ) For influent solids of 400 mg / L the difference in E for flows of 25 and 100 m 3 / m 2 . day is ;

DELTA E = { ( 0.955 ) exp - [ ( 265 / 400 ) + ( 0.0021 ) ( 25 ) ] } - { ( 0.955 ) exp - [ ( 265 / 400 ) + ( 0.0021 ) ( 100 ) ] } = 0.47 - 0.40 = 0.07 or 7 %

3.8. Activated Sludge Settlement...

The mixed liquor suspended solids ( MLSS ), as the mixture of tank solids is called, enters the final settlement tank as part of a continuous process. There is an underflow of thickened sludge which is returned to be mixed with fresh sewage. In the activated sludge settlement tanks, two processes take place ;

( a ) The clarification of the liquor ( effluent ) which goes over the outlet weir
( b ) The thickening of the sludge prior to return to the aeration tank

If a sample of activated sludge is shaken in a wide, deep tube ( say 1 m x 0.01 m ) it will settle with a distinct interface between sludge and liquor ( effluent ) and the plot of interface height versus time often forms a curve of the type shown in figure given below.



The percentage of the original volume of the column which the sludge occupies after 30 min settlement divided by the initial sludge concentration ( MLSS ) % gives the sludge volume index, SVI ;

SVI = ( Volume occupied by sludge as % of original volume ) / ( MLSS % )

This is used to give a qualitative estimate of the sludge settleability. The inverse of the SVI value multiplied by 100 gives the sludge density index ( SDI ). Values of 150 - 200 for the SVI are poor and values of <= 50 are good. From figure given above it can be seen that the rate of production of clarified effluent Q E is given by the product of the initial clarification velocity V I and the surface area A.

In a real tank given in figure shown below, there is an inlet flow ( Q E + q ) at concentration C O and two outflows ; ( a ) Q E with what is hopefully a negligible concentration of solids C E , and ( b ) an underflow q of solids concentration C U . The example below illustrates how the overflow rate concept may be used to determine a tank surface area.



Example 3-11 :

Assuming the sludge settlement curve given in figure shown above is representative of a mixed liquor of MLSS ( C O ) = 3,500 mg / L which is fed into an activated sludge settlement tank at 0.02 m 3 / s, calculate the tank surface area required for clarification if thickened sludge ise withdrawn at a concentration C U = 13,000 mg / L. Also calculate the SVI of the sludge.

Calculation :

From a mass balance ;

( Q E + q ) ( C O ) = ( Q E ) ( C E ) + ( q ) ( C U )

If C E is approximately equal to zero ( generally < 30 mg / L ) ;

( Q E / q ) = ( C U - C O ) / ( C O )

( Q E / q ) = ( 13,000 - 3,500 ) / ( 3,500 ) = 2.71

Q E = ( 2.71 / 3.71 ) ( 0.02 ) = 0.015 m 3 / s

( V I ) ( A ) = Q E

From the figure V I = 4.8 x 10 - 4 m / s

A REQUIRED = 0.015 / 4.8 x 10 - 4 = 31 m 2

Also, from the figure, the height of the sludge column after 30 min is 320 mm. The percentage settled volume after 30 min ;

[ ( A x 320 ) / ( A x 1,000 ) ] ( 100 ) = 32 %

The initial concentration C O = 3,500 mg / L which can be expressed as approximately 3,500 ppm ( parts per million ) since the density of the inflow is almost 1,000 kg / m 3 ;

C O = 0.35 % ( 10,000 ppm = 1 % )

SVI = ( 32 ) / ( 0.35 ) = 91

i.e. the sludge is of medium settleability.

3.9. Activated Sludge Thickening...

One of the most powerful theories relating to the thickening of activated sludges is that of flux thickening. It is based on the assumption that as a sludge thickens, i.e. changes its concentration C, the average velocity of that mass of sludge V is related to C by the expression ;

V = ( V 1 ) ( e - ( k ) ( C ) )

where ; V 1 and k : constants. In a batch settlement tank of area A the rate of transfer of solids downwards through a section " j " is equal to the product of V J and C J . The overall transfer of solids through the section " j " is given by ;

T S = ( V J ) ( C J ) + ( q x C / A ) ( j )

q / A is usually designated " U " being the underflow resultant velocity. If C E is negligible, then for a steady state, the input solids flux ;

[ ( Q E + q ) ( C O ) ] / ( A )

must equal T S . If the input flux is greater than T S , material will overflow, i.e. C E is not negligible. The effect of varying q / A is best seen by an example.

Example 3-12 :

If C is given as kg / m 3 and V is m / h ;

V = 6 e - 0.4 C

is a realistic description of the mass settling velocity of a particular activated sludge. Plot the V versus C curve and hence construct the flux settlement curve for underflow values ( q / A ) of 0.30 m / h and 0.80 m / h.

Calculation :

For C = 0 kg / m 3 , V = 6.00 m / h. For C = 1 kg / m 3 , V = 4.00 m / h.

Similarly, for various values of C, figure given below can be constructed.



Pairs of values for V and C can be calculated and the product V x C can be estimated and plotted. If the value of q / A or U is now chosen, a cumulative flux equal to ( V ) ( C ) + ( U ) ( C ) can also be determined and plotted as in figure given below.

C ( V ) ( C ) ( V ) ( C ) + ( U ) ( C )
( kg / m 3 ) ( kg / m 2 . h ) U = 0.30 m / h U = 0.80 m / h
0.0 0.0 0.0 0.0
2.0 5.4 6.0 7.0
4.0 4.8 6.1 8.1
6.0 3.3 5.1 8.1
8.0 2.0 4.4 8.4
10.0 1.1 4.1 9.1
12.0 0.6 4.2 10.2
14.0 0.3 4.5 11.5
16.0 0.16 5.0 13.0



If the value of q / A or U is now chosen, a cumulative flux equal to ( V ) ( C ) + ( U ) ( C ) can also be determined and plotted as in figure given below.



Considering curve " a " in the figure, i.e. U = 0.3 m / h, the compound flux curve shows a maximum at approximately C = 2.5 kg / m 3 and a minimum at C = 10.4 kg / m 3 . If the MLSS enters the tank at concentration C O = 4 kg / m 3 , then as the sludge thickens or increases in concentration, the ability of the tank to pass solids downwards diminishes until a value of 10.4 kg / m 3 is reached and subsequently increases. The minimum compound flux T S ( i.e. 4.1 kg / m 2 . h ) imposes a limit to the solids handling capacity of the thickener. If the solids concentration in the effluent stream, C E , is negligible, then the inlet solids flux, T S - I , must equal the return sludge solids flux, ( q / A ) ( C U ) unless there is storage or hold up of solids in the settlement tank. Where no hold up of solids occurs, i.e. ;

T S - I = [ ( Q E + q ) ( C O ) ] / ( A ) = ( q / A ) ( C U ) = ( U ) ( C U )

this relationship can be used to estimate C U .

Example 3-13 :

For curve " a " in figure given above, calculate the value of C U for inlet flux loadings of 4.1, 3.0 and 6.0 kg / m 2 . h.

Calculation :

For T S - I = T S - L = 4.1 kg / m 2 . h, this is equal to the solids handling capacity of the thickener and there should be no solids hold up ; thus ;

C U = T S - I / U = 4.1 / 0.3 = 13.7 kg / m 3

The other values can be calculated similarly. In this latter case the thickener is being overloaded at a rate of ( 6.0 - 4.1 ) kg / m 2 . h or 1.9 kg / m 2 . h. If these conditions persists eventually this amount of solids will be lost in the effluent stream. The condition may be relieved by increasing U. If U increased to 0.6 m / h, the value of T S - L increases to 6.7 kg / m 2 . h but now occurs at a concentration of 7.5 kg / m 3 . The value of C U for an inlet flux of 6.0 kg / m 2 . h then is 6.0 / 0.6 = 10 kg / m 3 . Now considering curve " b " in figure given above, as U attains the value of approximately 0.8 m / h there is merely a point of inflection in the combined flux curve, i.e. there is no minimum flux level T S - L . As before C U = T S - I / U ; however, the limiting criteria for the tank is now its role as a clarifier not as a thickener.

Example 3-14 :

Assuming V = 6 exp ( - 0.4 C ) describe the thickening behaviour of the sludge, shown by both general formulae and by calculation, that clarification is critical when U = 0.8 m / h ( approximately ).

Calculation :

If solids are not to be lost in the effluent stream ;

T S - I <= T S - L

Now ;

T S - I = [ ( Q E + q ) ( C O ) ] / ( A )

From figure given above, T S - L for U = 0.8 m / h has become in fact the value of T S at C = C O ( i.e. 8.1 kg / m 2 . h ).

T S - L = ( V I ) ( C O ) + ( q / A ) ( C O )

where V I is the initial settlement velocity of the solids at inlet concentration C O . By comparison of the above two expressions ;

V I >= Q E / A

which is the criterion for clarification as shown in section given above. When U = 0.8 m / h, an inlet flux of 8.1 kg / m 2 . h gives an underflow concentration C U of ;

C U = 8.1 / 0.8 = 10.1 kg / m 3

Therefore, for an inlet MLSS concentration of 4.0 kg / m 3

( Q E / q ) = ( C U - C O ) / ( C O ) = ( 10.1 - 4.0 ) / ( 4.0 ) = 1.5

and ( Q E / A ) = ( 1.5 ) ( 0.8 ) = 1.22 m / h. Thus, at a value of U = 0.8 m / h the effluent is being withdrawn at a rate equivalent to an upward flow velocity of 1.22 m / h for C O = 4.0 kg / m 3 . However, the initial settling velocity of the sludge bulk is given by ;

V I = 6 exp ( - 0.4 C O )

i.e. ;

1.21 m / h

i.e. the clarification rate criteria that the overflow velocity be less than the initial sludge settling velocity has been transgressed ( the velocities are actually equal for U = 0.79 m / h ).

Example 3-15 :

A works inflow of 0.10 m 3 / s produces an MLSS concentration of 4,000 mg / L from an activated sludge unit. The MLSS is to be thickened to 12,000 mg / L in a thickener. Calculate the area required and the loading rate if the sludge settling characteristics are described by the curve in figure given above. The settled sludge is to be returned to the inlet of the aeration tank.

Calculation :

The value of Q E is 0.10 m 3 / s or 360 m 3 / h, C U = 12 kg / m 3 and C O = 4 kg / m 3 . Therefore ;

( q / Q E ) = ( C O ) / ( C U - C O ) = 0.5

Thus, the return sludge flow is given by ;

q = ( 0.5 ) ( 360 ) = 180 m 3 / h

The actual inlet load is given by ;

( Q E + q ) ( C O ) = 2,160 kg / h

In figure given above, aligning a rule at C = 12 kg / m 3 to cut the curve tangentially, gives an ordinate value for T S - L of 5.8 kg / m 2 . h and a slope ( - U ) value of 5.8 / 12 = 0.48 m / h. Using this loading rate and letting A be the required area ;

T S - I <= T S - L

2,160 / A <= 5.8, i.e. A > 372 m 2

Using the underflow velocity as a check ;

( 180 / A ) = 0.48 then A = 375 m 2